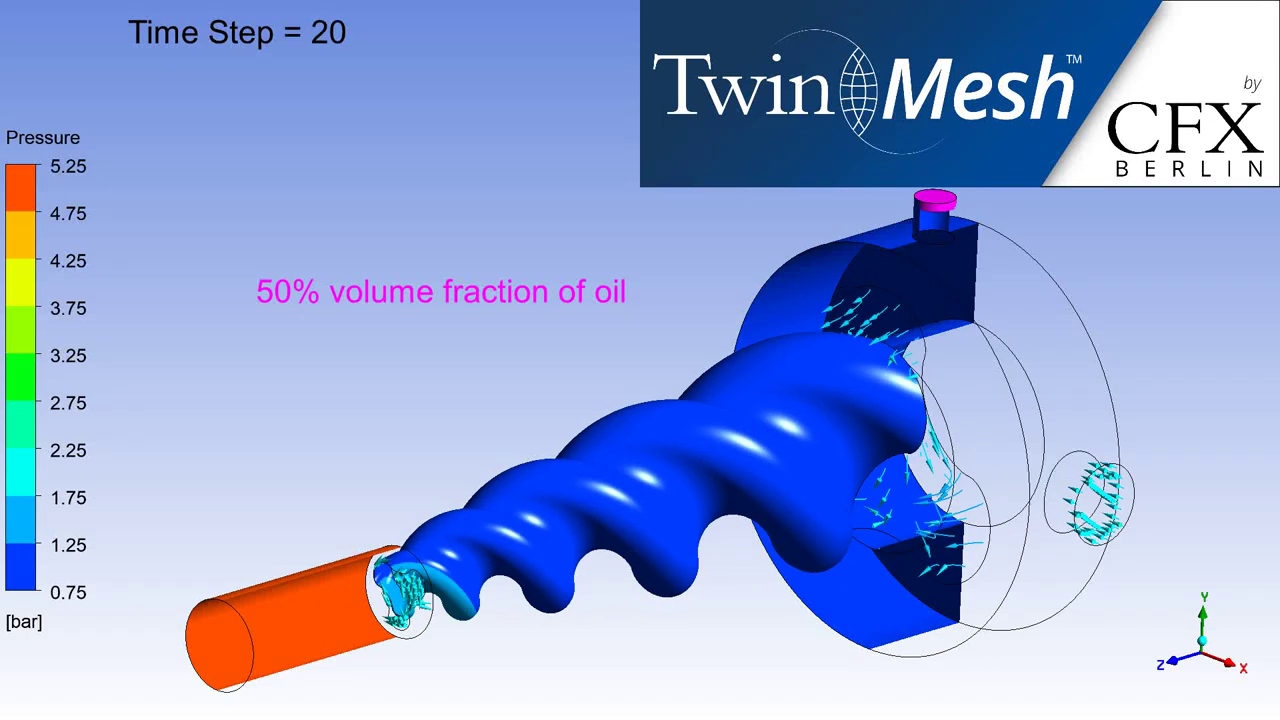
This animation shows a conical rotary compressor with non-parallel variable pitch rotors that compresses air from 1 bar to 5 bar. Starting from an initialization without oil, 3 l/min oil are injected. The oil seals the radial gap between both rotors, and cools down the gas during compression so that air flow increases compared to dry-running compressor. This simulation resembles that described in [1], but shapes of inner and outer rotor and inlet and outlet parts are chosen freely in the same ranges.
The shape of the conical rotary compressor is similar to a progressing cavity pump but with decreasing radial extent and decreasing chamber length in transport direction to compress the gas during transportation. The lobe combination of inner and outer rotor is 3 by 2. Outer rotor diameter is 80 mm at suction side, 18.56 mm at pressure side, the rotor length is 160 mm with a variable pitch, eccentricity at suction side 10 mm. The size of the radial gap between the rotors is 100 µm at suction side, decreasing proportionally to rotor diameters towards pressure side.
The meshes for the fluid region between both rotors were pre-generated for each 2° rotation angle of the inner rotor with TwinMesh. We used the outerfix approach, i.e. the mesh nodes are fixed on the outer rotor surface and move on the inner rotor surface. We discretized with 180 elements in circumferential direction, 25 elements in radial direction, and 180 elements in axial direction, i.e. with 810,000 hexahedrons for the rotating and deforming part. These meshes were transformed by Perl scripts to account for variable diameter and variable pitch angle along the z axis. Meshes for the static parts, i.e. suction and pressure side with air and oil injection pipes, were generated with Ansys Icem CFD and Ansys Meshing and consist of approx. 550,000 elements (mainly hexahedrons) together.
For the setup, we use air described as calorically perfect ideal gas, and incompressible oil with density 800 kg/m³. Boundary conditions are 1 bar and 20°C at air inlet and 5 bar at outlet with standard reflecting boundary conditions; at oil inlet 3 l/min oil enter at 20°C. The inner rotor rotates at 3000 rpm, the outer rotor at 2000 rpm, rotor walls are at 20°C. The multi-phase simulation starts from an initialization with 1 bar, 20°C, zero velocity and gas everywhere; only the outlet pipe was initialized at 5 bar and 191°C; The connection between rotors and outlet pipe was defined as an initially closed conditional interface that opens when pressure at rotor side reaches 5 bar what is the case at time step 1624.
For the multi-phase simulation, we use a homogeneous model, i.e. gas and oil phase share a common velocity and turbulence (SST model is used) field; two separate energy equations are solved, but coupled by transfer terms to ensure a common temperature field. Phase distribution is solved via the volume-of-fluid method; the free surface model with interface compression level 2 allows the separation of mixed phases by a gradient sharpening.
Time step size is 111 µs (2° rotation angle at 3000 rpm), 8 coefficient loops are used at each time step with high resolution scheme for advection and first order backward Euler scheme in time. Simulation was running for 4860 time steps, i.e. 27 revolutions of the inner rotor, 18 of the outer rotor, on 3 cores local parallel with Ansys CFX, and needed approx. 9.4 days of simulation time, i.e. 8 hours for one inner rotor revolution. Finally, the average air mass flow is 2.9 g/s (147 l/min), power consumption is 820 W.
The animation shows pressure distribution as contour plot on the inner rotor and parts of the inlet and outlet regions; the pressure rise in the compressor from suction towards pressure side can be seen. Additionally, velocity at inlet, at suction and at discharge port is shown by coloured streamlines with a length of 1 ms, and isosurface for an oil volume fraction of 50% is added in purple, showing the transport of oil through the compressor.
[1] Design and analysis of conical rotary compressor, Y Lu et al 2022 IOP Conf. Ser.: Mater. Sci. Eng. 1267 012001